Mathematics/Calculuses

This diagram shows an approximation to an area under a curve. Credit: Dubhe.

Notation: let the symbol $\Delta$ represent change in.

Notation: let the symbol $d$ represent an infinitesimal change in.

Notation: let the symbol $\partial$ represent an infinitesimal change in one of more than one.

Def. significant "change in or effect on a situation or state" or a "result of a subtraction; sometimes the absolute value of this result" is called a difference.

Def. a result of an "operation of deducing one function from another according to some fixed law" is called a derivative.

Let

$y=f(x)$

be a function where values of $x$ may be any real number and values resulting in $y$ are also any real number.

$\Delta x$ is a small finite change in $x$ which when put into the function $f(x)$ produces a $\Delta y$.

These small changes can be manipulated with the operations of arithmetic: addition ($+$), subtraction ($-$), multiplication ($*$), and division ($/$).

$\Delta y=f(x+\Delta x)-f(x)$

Dividing $\Delta y$ by $\Delta x$ and taking the limit as $\Delta x$ → 0, produces the slope of a line tangent to f(x) at the point x.

For example,

$f(x)=x^{2}$

$f(x+\Delta x)=(x+\Delta x)^{2}=x^{2}+2x\Delta x+\Delta x^{2}$

$\Delta y=x^{2}+2x\Delta x+\Delta x^{2}-x^{2}=2x\Delta x+\Delta x^{2}$

${\Delta y \over \Delta x}={2x\Delta x+\Delta x^{2} \over \Delta x}=2x+\Delta x$

as $\Delta x$ and$\Delta y$ go towards zero,

$dy/dx=2x+dx=limit_{\Delta x\to 0}{f(x+\Delta x)-f(x) \over \Delta x}=2x.$

This ratio is called the derivative.

Let

$y=f(x,z)$

then

$\partial y=\partial f(x,z)=\partial f(x,z)\partial x+\partial f(x,z)\partial z$

$\partial y/\partial x=\partial f(x,z)$

where z is held constant and

$\partial y/\partial z=\partial f(x,z)$

where x is held contstant.

Notation: let the symbol $\nabla$ be the gradient, i.e., derivatives for multivariable functions.

$\nabla f(x,z)=\partial y=\partial f(x,z)=\partial f(x,z)\partial x+\partial f(x,z)\partial z.$

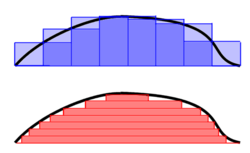
For

$\Delta x*\Delta y=[f(x+\Delta x)-f(x)]*\Delta x$

the area under the curve shown in the diagram at right is the light purple rectangle plus the dark purple rectangle in the top figure

$\Delta x*\Delta y+f(x)*\Delta x=f(x+\Delta x)*\Delta x.$

Any particular individual rectangle for a sum of rectangular areas is

$f(x_{i}+\Delta x_{i})*\Delta x_{i}.$

The approximate area under the curve is the sum $\sum$ of all the individual (i) areas from i = 0 to as many as the area needed (n):

$\sum _{i=0}^{n}f(x_{i}+\Delta x_{i})*\Delta x_{i}.$

Notation: let the symbol $\int$ represent the integral.

$limit_{\Delta x\to 0}\sum _{i=0}^{n}f(x_{i}+\Delta x_{i})*\Delta x_{i}=\int f(x)dx.$

This can be within a finite interval [a,b]

$\int _{a}^{b}f(x)\;dx$

when i = 0 the integral is evaluated at $a$ and i = n the integral is evaluated at $b$. Or, an indefinite integral (without notation on the integral symbol) as n goes to infinity and i = 0 is the integral evaluated at x = 0.

Def. a branch of mathematics that deals with the finding and properties of infinitesimal differences or changes is called a calculus.

Calculus focuses on limits, functions, derivatives, integrals, and infinite series.

"Although calculus (in the sense of analysis) is usually synonymous with infinitesimal calculus, not all historical formulations have relied on infinitesimals (infinitely small numbers that are nevertheless not zero)."

External links

{{Reasoning resources}}