Telescopes and cameras

Diagram of the focal ratio of a simple optical system where $f$ is the focal length and $D$ is the diameter of the objective lens. Credit: Vargklo.{{free media}}

In most photography and all telescopy, where the subject is essentially infinitely far away, longer focal length (lower optical power) leads to higher magnification and a narrower angle of view; conversely, shorter focal length or higher optical power is associated with a wider angle of view.

Problem 1

This is a double-convex, thick lens diagram. Credit: Tamasflex.{{free media}}

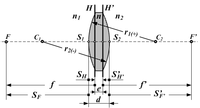
For the case of a [double-convex] lens of thickness d in air, and surfaces with radii of curvature R₁ and R₂, the effective focal length f is given by:

${\frac {1}{f}}=(n-1)\left[{\frac {1}{R_{1}}}-{\frac {1}{R_{2}}}+{\frac {(n-1)d}{nR_{1}R_{2}}}\right],$

where n is the refractive index of the lens medium. The quantity 1/f is also known as the optical power of the lens.

What is the formula for a plano-convex lens?

Calculate the focal length of a double convex lens in which R₁ = 10 m and R₂ = 200 m. Let n = 1.732 and d = 2 cm.

What is the focal length of a hemispherical plano-convex lens of radius 10 m? Let n= 1.324 and d = 1 dm.

What is a likely focal length for a plano-convex lens where the convex surface is parabolic rather than spherical?

Problem 2

Diagram of decreasing apertures, that is, increasing f-numbers, in one-stop increments; each aperture has half the light gathering area of the previous one. Credit: Cbuckley.{{free media}}

For a focal length of 100 mm, what are the aperture or entrance pupil diameters for each of the apertures shown if no optical components are between the entrance pupil and the aperture?

For a simple camera or telescope with an aperture lens diameter of 5 m, what is the focal length for each of the apertures or entrance pupil diameters in the diagram if no optical components are between the entrance pupil and the aperture?

For the following pairs of focal lengths and lens diameters, what would be the f-numbers? (a) 5 m, 3 m (b) 10 m, 3 mm (c) 50 cm, 2 m (d) 15 km, 25 m (e) 5,000 km, 16 cm.

Problem 3

A 35 mm lens is set to $f/11$, as indicated by the white dot above the f-stop scale on the aperture ring. Credit: MarkSweep.{{free media}}

The lens in the image at right has an aperture range of $f/2.0$ to $f/22.$

The image lens at right uses a standard f-stop scale, which is an approximately geometric sequence of numbers that corresponds to the sequence of the powers of the square root of 2:   $f/1,$ $f/1.4$ $f/2,$ $f/2.8,$ $f/4,$ $f/5.6,$ $f/8,$ $f/11,$ $f/16,$ [and] $f/22.$

The sequence above is obtained by approximating the following exact geometric sequence:

$f/1={\frac {f/1}{({\sqrt {2}})^{0}}},$

$f/1.4={\frac {f/1}{({\sqrt {2}})^{1}}},$

$f/2={\frac {f/1}{({\sqrt {2}})^{2}}},$

$f/2.8={\frac {f/1}{({\sqrt {2}})^{3}}},$

$f/4=,$

$f/5.6=,$

$f/8=,$

$f/11=,$

$f/16=,$

$f/22=.$

What are the missing terms?

Each "stop" is marked with its corresponding f-number, and represents a halving of the light intensity from the previous stop. This corresponds to a decrease of the pupil and aperture diameters by a factor of 1/$\scriptstyle {\sqrt {2}}$ or about 0.7071, and hence a halving of the area of the pupil.

For a lens diameter of 35 mm, what is the focal length for each f-stop?

Problem 4

Shutter speeds are arranged so that each setting differs in duration by a factor of approximately two from its neighbour. Opening up a lens by one stop allows twice as much light to fall on the film in a given period of time. Therefore to have the same exposure at this larger aperture as at the previous aperture, the shutter would be opened for half as long (i.e., twice the speed). The film will respond equally to these equal amounts of light, since it has the property of reciprocity. This is less true for extremely long or short exposures, where we have reciprocity failure. Aperture, shutter speed, and film sensitivity are linked: for constant scene brightness, doubling the aperture area (one stop), halving the shutter speed (doubling the time open), or using a film twice as sensitive, has the same effect on the exposed image.

Shutter speed or exposure time is the length of time a camera's shutter is open when taking a photograph. The amount of light that reaches the film or image sensor is proportional to the exposure time.

Exposure value (EV) is a single quantity that accounts for the shutter speed and the f-number.

Multiple combinations of shutter speed and f-number can give the same exposure value.

Doubling the exposure time doubles the amount of light (subtracts 1 EV). Making the f-number one stop brighter (reducing the f-number by a factor of $\scriptstyle {\sqrt {2}}$) also doubles the amount of light. A shutter speed of 1/50 s with an $f/4.0$ lens gives the same exposure value as a 1/100 s shutter with an {$f/2.8$ lens, and also the same exposure value as a 1/200 s shutter with an $f/2.0$ lens.

A standardized 2:1 scale for shutter speed is such that opening one aperture stop and reducing the shutter speed by one step resulted in the identical exposure. The standards for shutter speeds are:

• 1/16000 s
• 1/12000 s
• 1/8000 s
• 1/4000 s
• 1/2000 s
• 1/1000 s
• 1/500 s
• 1/250 s
• 1/125 s
• 1/60 s
• 1/30 s
• 1/15 s
• 1/8 s
• 1/4 s
• 1/2 s
• 1 s

What are the other f-numbers that match the shutter speeds?

Exposure value (EV) is a number that represents a combination of a camera's shutter speed and f-number, such that all combinations that yield the same exposure have the same EV value (for any fixed scene luminance). Exposure value also is used to indicate an interval on the photographic exposure scale, with 1 EV corresponding to a standard power-of-2 exposure step, commonly referred to as a stop.

Exposure value is a base-2 logarithmic scale:

$\mathrm {EV} =\log _{2}{\frac {N^{2}}{t}}\,,$

where

• N is the relative aperture (f-number)
• t is the exposure time (“shutter speed”) in seconds.

In a mathematical expression involving physical quantities, it is common practice to require that the argument to a transcendental function (such as the logarithm) be dimensionless. The definition of EV ignores the units in the denominator and uses only the numerical value of the exposure time in seconds; EV is not the expression of a physical law, but simply a number for encoding combinations of camera settings.

Match up the shutter speeds with f-numbers to give the same EV.

Hypotheses

Main resource: Hypotheses

1. Alternate title could be Radiation telescopes/Problem set.

See also

• Angular momentum and energy
• Column densities
• Cosmic circuits
• Energy phantoms
• Furlongs per fortnight
• Planck's equation
• Radiation astronomy/Problem set
• Radiation dosage
• Radiation astromathematics problems
• Spectrographs
• Star jumping
• Synchrotron radiation
• Unknown coordinate systems
• Unusual units
• Vectors and coordinates

External links

• International Astronomical Union
• NASA/IPAC Extragalactic Database - NED
• NASA's National Space Science Data Center
• NCBI All Databases Search
• Office of Scientific & Technical Information
• The SAO/NASA Astrophysics Data System
• Scirus for scientific information only advanced search
• SDSS Quick Look tool: SkyServer
• SIMBAD Astronomical Database
• SIMBAD Web interface, Harvard alternate
• Spacecraft Query at NASA
• Universal coordinate converter

{{Archaeology resources}}

{{Radiation astronomy resources}}{{Reasoning resources}}{{Technology resources}}