WikiJournal Preprints/Huygens' principle

From Wikiversity
Jump to navigation Jump to search

WikiJournal Preprints
Open access • Publication charge free • Public peer review

Submit
Authors
Reviewers
Editors
About

WikiJournal User Group is a publishing group of open-access, free-to-publish, Wikipedia-integrated academic journals. <seo title=" Wikiversity Journal User Group, WikiJournal Free to publish, Open access, Open-access, Non-profit, online journal, Public peer review "/>

<meta name='citation_doi' value=>

Article information

Author: Gavin R. Putland[i]  

See author information ▼
  1. ↑ Contact form

Abstract

Nearly all of the traffic to the Wikipedia article "Huygens–Fresnel principle" is apparently redirected from "Huygens' Principle" (not "Huygens's…", which gets nearly zero hits). Accordingly I submit that the article should be split into two: "Huygens' principle" and "Huygens–Fresnel principle". In this first version of the former (not really a draft), I have done little but remove material peculiar to Fresnel.


Wave refraction in the manner of Huygens
Wave diffraction in the manner of Huygens and Fresnel

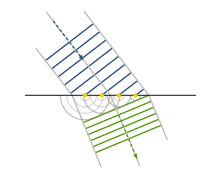
Huygens' principle (named after Dutch physicist Christiaan Huygens) states that every point reached by a traveling wave is itself the source of a secondary wave.[1] The superposition of these secondary waves forms a new primary wavefront. The principle is applicable to problems of luminous wave propagation both in the far-field limit and in near-field diffraction as well as reflection.

History[edit | edit source]

Diffraction of a plane wave when the slit width equals the wavelength

In 1678, Huygens proposed that every point reached by a luminous disturbance becomes a source of a spherical wave; the sum of these secondary waves determines the form of the wave at any subsequent time.[2] He assumed that the secondary waves travelled only in the "forward" direction and it is not explained in the theory why this is the case. He was able to provide a qualitative explanation of linear and spherical wave propagation, and to derive the laws of reflection and refraction using this principle, but could not explain the deviations from rectilinear propagation that occur when light encounters edges, apertures and screens, commonly known as diffraction effects.[3] The resolution of this error was finally explained by David A. B. Miller in 1991.[4] The resolution is that the source is a dipole (not the monopole assumed by Huygens), which cancels in the reflected direction.

In 1818, Fresnel[5] showed that Huygens's principle, together with his own principle of interference could explain both the rectilinear propagation of light and also diffraction effects. To obtain agreement with experimental results, he had to include additional arbitrary assumptions about the phase and amplitude of the secondary waves, and also an obliquity factor (see Huygens–Fresnel principle). These assumptions have no obvious physical foundation but led to predictions that agreed with many experimental observations, including the Poisson spot.

Poisson was a member of the French Academy, which reviewed Fresnel's work.[6] He used Fresnel's theory to predict that a bright spot ought to appear in the center of the shadow of a small disc, and deduced from this that the theory was incorrect. However, Arago, another member of the committee, performed the experiment and showed that the prediction was correct. (Lisle had observed this fifty years earlier.[3][dubious – discuss]) This was one of the investigations that led to the victory of the wave theory of light over then predominant corpuscular theory.

In antenna theory and engineering, the reformulation of Huygens' principle for radiating current sources is known as the surface equivalence principle[7][8] (or Huygens' equivalence principle).

Huygens' principle as a microscopic model[edit | edit source]

Huygens' principle provides a reasonable basis for understanding and predicting the classical wave propagation of light. However, there are limitations to the principle, namely the same approximations done for deriving the Kirchhoff's diffraction formula and the approximations of near field due to Fresnel. These can be summarized in the fact that the wavelength of light is much smaller than the dimensions of any optical components encountered.[6]

Kirchhoff's diffraction formula provides a rigorous mathematical foundation for diffraction, based on the wave equation. The ad-hoc assumptions made by Fresnel to arrive at the Huygens–Fresnel equation[clarification needed] emerge automatically from the mathematics in this derivation.[9]

A simple example of the operation of the principle can be seen when an open doorway connects two rooms and a sound is produced in a remote corner of one of them. A person in the other room will hear the sound as if it originated at the doorway. As far as the second room is concerned, the vibrating air in the doorway is the source of the sound.

Modern physics interpretations[edit | edit source]

Not all experts agree that the Huygens' principle is an accurate microscopic representation of reality. For instance, Melvin Schwartz argued that "Huygens' principle actually does give the right answer but for the wrong reasons".[1]

This can be reflected in the following facts:

  • The microscopic mechanics to create photons and of emission, in general, is essentially acceleration of electrons.[1]
  • The original analysis of Huygens[10] included amplitudes only. It includes neither phases nor waves propagating at different speeds (due to diffraction within continuous media), and therefore does not take into account interference.
  • The Huygens analysis also does not include polarization for light which imply a vector potential, where instead sound waves can be described with a scalar potential and there is no unique and natural translation between the two.[11]
  • In the Huygens description, there is no explanation of why we choose only the forward-going (retarded wave or forward envelope of wave fronts) versus the backward-propagating advanced wave (backward envelope).[11]
  • The principle is non-local in that it combines secondary waves coming from different parts of the primary wave, with different delays. Non-local theories are the subject of many debates (e.g., for not being Lorentz-covariant) and of active research.[citation needed]
  • While the addition of secondary wave functions can be interpreted in a quantum probabilistic manner, it is unclear how much this represents a complete list of states that are meaningful physically, or an approximation on a generic basis as in the linear combination of atomic orbitals (LCAO) method.

The Huygens' principle is essentially compatible with quantum field theory in the far field approximation, considering effective fields in the center of scattering, considering small perturbations, and in the same sense that quantum optics is compatible with classical optics, other interpretations are subject of debates and active research.

The Feynman model where every point in an imaginary wave front as large as the room is generating a wavelet, shall also be interpreted in these approximations [12] and in a probabilistic context, in this context remote points can only contribute minimally to the overall probability amplitude.

Quantum field theory does not include any microscopic model for photon creation and the concept of single photon is also put under scrutiny on a theoretical level.

Generalized Huygens' principle[edit | edit source]

Many books and references e.g.[13] and [14] refer to the Generalized Huygens' Principle as the one referred by Feynman in this publication.[15]

Feynman defines the generalized principle in the following way:

Actually Huygens' principle is not correct in optics. It is replaced by Kirchoff's [sic] modification which requires that both the amplitude and its derivative must be known on the adjacent surface. This is a consequence of the fact that the wave equation in optics is second order in the time. The wave equation of quantum mechanics is first order in the time; therefore, Huygens' principle is correct for matter waves, action replacing time.

This clarifies the fact that in this context the generalized principle reflects the linearity of quantum mechanics and the fact that the quantum mechanics equations are first order in time. Finally only in this case the superposition principle fully apply, i.e. the wave function in a point P can be expanded as a superposition of waves on a border surface enclosing P. Wave functions can be interpreted in the usual quantum mechanical sense as probability densities where the formalism of Green's functions and propagators apply. What is note-worthy is that this generalized principle is applicable for "matter waves" and not for light waves any more. The phase factor is now clarified as given by the action and there is no more confusion why the phases of the wavelets are different from the one of the original wave and modified by the additional Fresnel parameters.

As per Greiner [13] the generalized principle can be expressed for in the form:

Where G is the usual Green function that propagates in time the wave function . This description resembles and generalize the initial Fresnel's formula of the classical model.

Huygens' theory, Feynman's path integral and the modern photon wave function[edit | edit source]

Huygens' theory served as a fundamental explanation of the wave nature of light interference and was further developed by Fresnel and Young but did not fully resolve all observations such as the low-intensity double-slit experiment first performed by G. I. Taylor in 1909. It was not until the early and mid-1900s that quantum theory discussions, particularly the early discussions at the 1927 Brussels Solvay Conference, where Louis de Broglie proposed his de Broglie hypothesis that the photon is guided by a wave function.[16]

The wave function presents a much different explanation of the observed light and dark bands in a double slit experiment. In this conception, the photon follows a path which is a probabilistic choice of one of many possible paths in the electromagnetic field. These probable paths form the pattern: in dark areas, no photons are landing, and in bright areas, many photons are landing. The set of possible photon paths is consistent with Richard Feynman's path integral theory, the paths determined by the surroundings: the photon's originating point (atom), the slit, and the screen and by tracking and summing phases. The wave function is a solution to this geometry. The wave function approach was further supported by additional double-slit experiments in Italy and Japan in the 1970s and 1980s with electrons.[17]

Huygens' principle and quantum field theory[edit | edit source]

Huygens' principle can be seen as a consequence of the homogeneity of space—space is uniform in all locations.[18] Any disturbance created in a sufficiently small region of homogeneous space (or in a homogeneous medium) propagates from that region in all geodesic directions. The waves produced by this disturbance, in turn, create disturbances in other regions, and so on. The superposition of all the waves results in the observed pattern of wave propagation.

Homogeneity of space is fundamental to quantum field theory (QFT) where the wave function of any object propagates along all available unobstructed paths. When integrated along all possible paths, with a phase factor proportional to the action, the interference of the wave-functions correctly predicts observable phenomena. Every point on the wavefront acts as the source of secondary wavelets that spread out in the light cone with the same speed as the wave. The new wavefront is found by constructing the surface tangent to the secondary wavelets.

In other spatial dimensions[edit | edit source]

In 1900, Jacques Hadamard observed that Huygens' principle was broken when the number of spatial dimensions is even.[19][20][21] From this, he developed a set of conjectures that remain an active topic of research.[22][23] In particular, it has been discovered that Huygens' principle holds on a large class of homogeneous spaces derived from the Coxeter group (so, for example, the Weyl groups of simple Lie algebras).[18][24]

The traditional statement of Huygens' principle for the D'Alembertian gives rise to the KdV hierarchy; analogously, the Dirac operator gives rise to the AKNS hierarchy.[25][26]

Additional information[edit | edit source]

Acknowledgments[edit | edit source]

As of 17 September 2023, this article is almost entirely the work of the Wikipedia community.

Competing interests[edit | edit source]

None.

Ethics statement[edit | edit source]

This article does not concern research on human or animal subjects.

See also[edit | edit source]

Template:Div-col

Template:Div-col-end

Notes[edit | edit source]

References[edit | edit source]

  1. ↑ 1.0 1.1 1.2 "Huygens' Principle". MathPages. Retrieved 2017-10-03.
  2. ↑ Chr. Huygens, Traité de la Lumière (drafted 1678; published in Leyden by Van der Aa, 1690), translated by Silvanus P. Thompson as Treatise on Light (London: Macmillan, 1912; Project Gutenberg edition, 2005), p.19.
  3. ↑ 3.0 3.1 Heavens, O. S.; Ditchburn, R. W. (1987). Insight into Optics. Chichester: Wiley & Sons. ISBN 0-471-92769-4. 
  4. ↑ Miller, David A. B. (1991). "Huygens's wave propagation principle corrected". Optics Letters 16 (18): 1370–1372. doi:10.1364/OL.16.001370. PMID 19776972. https://semanticscholar.org/paper/0dd2d800622bbcf22472c1c8042f11d390bb9ea5. 
  5. ↑ A. Fresnel, "Mémoire sur la diffraction de la lumière" (deposited 1818, "crowned" 1819), in Oeuvres complètes (Paris: Imprimerie impériale, 1866–70), vol.1, pp. 247–363; partly translated as "Fresnel's prize memoir on the diffraction of light", in H. Crew (ed.), The Wave Theory of Light: Memoirs by Huygens, Young and Fresnel, American Book Co., 1900, pp. 81–144. (Not to be confused with the earlier work of the same title in Annales de Chimie et de Physique, 1:238–81, 1816.)
  6. ↑ 6.0 6.1 Born, Max; Wolf, Emil (1999). Principles of Optics. Cambridge University Press. ISBN 978-0-521-64222-4. 
  7. ↑ Balanis, Constantine A. (2012). Advanced Engineering Electromagnetics. John Wiley & Sons. pp. 328–331. ISBN 978-0-470-58948-9. 
  8. ↑ Balanis, Constantine A. (2005). Antenna Theory: Analysis and Design (3rd ed.). John Wiley and Sons. p. 333. ISBN 047166782X. 
  9. ↑ Klein, M. V.; Furtak, T. E. (1986). Optics (2nd ed.). New York: John Wiley & Sons. ISBN 0-471-84311-3. 
  10. ↑ "Huygens". Archive.org. Retrieved 2020-07-02.
  11. ↑ 11.0 11.1 "TheoryOfHuygens". Archive.org. 1939.
  12. ↑ "Los Alamos Science". 2002.
  13. ↑ 13.0 13.1 Greiner W.. Quantum Electrodynamics. Springer, 2002. 
  14. ↑ Enders, Peter (2009). "Huygens' Principle as Universal Model of Propagation". Latin-American Journal of Physics Education 3 (1): 19–32. http://lajpe.org/jan09/04_Peter_Enders.pdf. 
  15. ↑ Feynman, R. P. (1 April 1948). "Space-Time Approach to Non-Relativistic Quantum Mechanics". Reviews of Modern Physics 20 (2): 367–387. doi:10.1103/RevModPhys.20.367. https://resolver.caltech.edu/CaltechAUTHORS:20140731-165931911. 
  16. ↑ Baggott, Jim (2011). The Quantum Story. Oxford Press. p. 116. ISBN 978-0-19-965597-7. https://archive.org/details/quantumstoryhist00bagg. 
  17. ↑ Peter, Rodgers (September 2002). "The double-slit experiment". www.physicsworld.com. Physics World. Retrieved 10 Sep 2018.
  18. ↑ 18.0 18.1 Veselov, Alexander P. (1995). "Huygens' principle and integrable systems". Physica D: Nonlinear Phenomena 87 (1–4): 9–13. doi:10.1016/0167-2789(95)00166-2. 
  19. ↑ Veselov, Alexander P. (2002). "Huygens' Principle" (PDF). Archived from the original (PDF) on 2016-02-21.
  20. ↑ "Wave Equation in Higher Dimensions" (PDF). Math 220a class notes. Stanford University.
  21. ↑ Belger, M.; Schimming, R.; Wünsch, V. (1997). "A Survey on Huygens' Principle". Zeitschrift für Analysis und ihre Anwendungen 16 (1): 9–36. doi:10.4171/ZAA/747. 
  22. ↑ Ásgeirsson, Leifur (1956). "Some hints on Huygens' principle and Hadamard's conjecture". Communications on Pure and Applied Mathematics 9 (3): 307–326. doi:10.1002/cpa.3160090304. 
  23. ↑ Günther, Paul (1991). "Huygens' principle and Hadamard's conjecture". The Mathematical Intelligencer 13 (2): 56–63. doi:10.1007/BF03024088. 
  24. ↑ Berest, Yu. Yu.; Veselov, A. P. (1994). "Hadamard's problem and Coxeter groups: New examples of Huygens' equations". Functional Analysis and Its Applications 28 (1): 3–12. doi:10.1007/BF01079005. 
  25. ↑ Chalub, Fabio A. C. C.; Zubelli, Jorge P. (2006). "Huygens' Principle for Hyperbolic Operators and Integrable Hierarchies". Physica D: Nonlinear Phenomena 213 (2): 231–245. doi:10.1016/j.physd.2005.11.008. 
  26. ↑ Berest, Yuri Yu.; Loutsenko, Igor M. (1997). "Huygens' Principle in Minkowski Spaces and Soliton Solutions of the Korteweg-de Vries Equation". Communications in Mathematical Physics 190 (1): 113–132. doi:10.1007/s002200050235. 

Bibliography[edit | edit source]

  • M. Born and E. Wolf, 2002, Principles of Optics, 7th Ed., Cambridge, 1999 (reprinted with corrections, 2002).
  • O. Darrigol, 2012, A History of Optics: From Greek Antiquity to the Nineteenth Century, Oxford.
  • F.J. Dijksterhuis, 1999, Lenses and Waves: Christiaan Huygens and the Mathematical Science of Optics in the Seventeenth Century  (doctoral thesis),  University of Twente;  doc.utwente.nl/33764. (See also the book with the same author and title,  Dordrecht: Kluwer Academic Publishers, 2004.)
  • F.J. Dijksterhuis, 2004, "Once Snell breaks down: From geometrical to physical optics in the seventeenth century", Annals of Science, vol. 61, no. 2 (Apr. 2004), pp. 165–85.
  • E. Hecht, 2017, Optics, 5th (Global) Ed., Pearson Education.
  • F.A. Jenkins and H.E. White, 1976, Fundamentals of Optics, 4th Ed., New York: McGraw-Hill.
  • I. Newton (2010), Opticks: or, a Treatise of the Reflections, Refractions, Inflections, and Colours of Light, 4th Ed. (first published London: William Innys, 1730), Mineola, NY: Dover, 1952, 1979, 2012; Project Gutenberg, 2010, gutenberg.org/ebooks/33504. (Cited page numbers match the Gutenberg HTML editions and the Dover editions.)
  • J.A. Stratton, 1941, Electromagnetic Theory, New York: McGraw-Hill.

Further reading[edit | edit source]

  • B.B. Baker and E.T. Copson, The Mathematical Theory of Huygens' Principle, Oxford, 1939, 1950; AMS Chelsea, 1987.
Retrieved from "https://en.wikiversity.org/w/index.php?title=WikiJournal_Preprints/Huygens%27_principle&oldid=2570298"