Physics equations/Static forces
Appearance
Most applications of Newton's laws require only a few equations
[edit | edit source]
Friction and the normal force
[edit | edit source]- is the force friction when an object is sliding on a surface, where ("mew-sub-k") is the kinetic coefficient of friction, and N is the normal force.
- establishes the maximum possible friction (called static friction) that can occur before the object begins to slide. Usually .
Also, air drag often depends on speed, an effect this model fails to capture.
These equations for static and kinetic friction almost always are valid only as approximations.
*** Problem: Generating equations from adding forces
[edit | edit source]*** Problem: Almost proving Netwon's third law (one dimensional)
[edit | edit source]*** Problem: A free-body diagram on a system with many parts
[edit | edit source]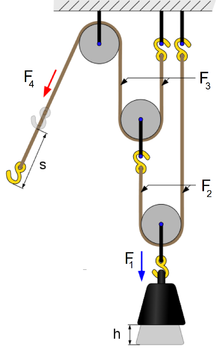 |
Make free body diagrams for each component of this system. Include attachments to the ceiling. |























