University of Florida/Egm4313/IEA-f13-team10/R5

Report 5

Problem 1: Taylor Series Expansion of the log Function

Problem Statement

$y''+6y'+9y=log(3+4x)$
$y(0)=1,y'(0)=.5$
Use the point
${\widehat {x}}=-1/2$
$\log x=\log _{e}x=\ln x$

Solution

$f(x)=\log(x)$
$f({\widehat {x}})=\log({\widehat {x}})$

$\sum _{k=0}^{\infty }{\frac {(f^{k})({\widehat {x}})}{k!}}(x-{\widehat {x}})^{k}$
$f^{1}(x)={\frac {1}{1+x}}=f^{1}({\widehat {x}})={\frac {1}{1+({\widehat {x}})}}$
Set
$w(x)=3+4x$
$w'(x)=4$

$f^{2}(x)={\frac {-1*w'*4}{w^{2}}}={\frac {-1}{w^{2}}}$

$f^{3}(x)={\frac {1*2*w*w'*4^{2}}{w^{4}}}={\frac {1*2}{w^{3}}}$

$f^{4}(x)={\frac {-1*2*3*w^{2}*w'*4^{3}}{w^{6}}}={\frac {-1*2*3}{w^{4}}}$

$f^{k}(x)={\frac {(-1)^{k-1}*(k-1)!*4^{k-1}}{w^{k}}}$

$\sum _{k=0}^{\infty }{\frac {(f^{k})({\widehat {x}})}{k!}}(x-{\widehat {x}})^{k}=\sum _{k=0}^{\infty }{\frac {(-1)^{k-1}(k-1)!}{k!(1+({\widehat {x}}))^{k}}}(x-{\widehat {x}})^{k}$

For $log(3+4x)$ the series expansion results in,
$\sum _{k=0}^{\infty }(-1)^{k+1}*({\frac {(2+4x)^{k}}{k}})$

Plots of taylor series expansion: Up to order 4


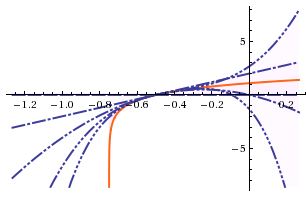
Up to order 7


Up to order 11


Up to order 16


The visually estimated domain of convergence is from .8 to .2.
Now use the transformation of variable
$x\longrightarrow t\ {\text{ such that }}\ 3+4x=1+t$
$x={\frac {t-2}{4}}$

If $log(1+t)$ has a domain of convergence from $[-1,+1]$ then $log(3+4x)$ converges from $[{\frac {-3}{4}},{\frac {-1}{4}}]$

Honor Pledge

On our honor, we solved this problem on our own, without aid from online solutions or solutions from previous semesters.

Problem 2: Plots of Truncated Series

Number 1

Plot at least 3 truncated series to show convergence

$\sum _{m=0}^{inf}{\frac {x^{2m+1}}{(2m+1)!}}$

m=0:$x$

m=1:$x+{\frac {x^{3}}{3*2*1}}$

m=2:$x+{\frac {x^{3}}{3*2*1}}+{\frac {x^{5}}{5*4*3*2*1}}$

Number 2

Plot at least 3 truncated series to show convergence

$\sum _{m=0}^{inf}({\frac {2}{3}})^{m}x^{2}m$

m=0:$x$

m=1:$x+{\frac {2}{3}}x^{2}$

m=2:$x+{\frac {2}{3}}x^{2}+{\frac {4}{9}}x^{4}$

Number 3

Find the radius of convergence for the taylor series of sinx, x = 0

The Taylor series of sinx is:

$\sum _{m=0}^{inf}{\frac {(-1)^{m}(x)^{2m+1}}{(2m+1)!}}$

The radius of convergence can be found by:

$R_{c}=[lim\left|{\frac {d_{k+1}}{d_{k}}}\right|]^{-1}$

$R_{c}=[lim{\frac {(-1)^{m+1}}{(2m+3)!}}*{\frac {(2m+1)!}{(-1)^{m}}}]^{-1}$

$R_{c}=\infty$

Number 4

Find the radius of convergence for the taylor series of log(1+x), x = 0

The Taylor series of log(x+1) is:

$-\sum _{n=1}^{\infty }{\frac {(-1)^{n}(x)^{n}}{n}}$

The radius of convergence can be found by:

$R_{c}=[lim\left|{\frac {d_{k+1}}{d_{k}}}\right|]^{-1}$

$R_{c}=[lim{\frac {(-1)^{n+1}}{n+1}}*{\frac {n}{(-1)^{n}}}]^{-1}$
$R_{c}=1$

Number 5

Find the radius of convergence for the taylor series of log(1+x), x = 1

The Taylor series of log(x+1) is:

$-\sum _{n=1}^{\infty }{\frac {(-1)^{n}(x-1)^{n}}{n}}$

The radius of convergence can be found by:

$R_{c}=[lim\left|{\frac {d_{k+1}}{d_{k}}}\right|]^{-1}$

$R_{c}=[lim{\frac {(-1)^{n+1}}{n+1}}*{\frac {n}{(-1)^{n}}}]^{-1}$

$R_{c}=1$

Number 6

derive the expression for the radius of convergence of log(1+x) about any focus point

The taylor series of log(1+x) is:

$-\sum _{n=1}^{\infty }{\frac {(-1)^{n}(x-{\widehat {x}})^{n}}{n}}$

$R_{c}=[{\frac {(-1)^{n+1}({\widehat {x}})^{n+1}}{n+1}}*{\frac {n}{(-1)^{n}({\widehat {x}})^{n}}}]^{-1}$

Number 7

Find the Taylor series representation of log(3+4x)

$\sum _{k=0}^{\infty }{\frac {(f)^{k}({\widehat {x}})}{k!}}*(x-{\widehat {x}})^{k}$
Expanding out 4 terms results in,
[ $r(x)=0+{\frac {4*(x-{\widehat {x}})}{3+4{\widehat {x}}}}-{\frac {(4^{2})*((x-{\widehat {x}})^{2}}{((3+4{\widehat {x}})^{2})*(2!)}}+{\frac {2(4^{2})*((x-{\widehat {x}})^{3}}{((3+4{\widehat {x}})^{3})*(3!)}}$
The series representation is
$\sum _{k=0}^{\infty }(-1)^{k+1}*({\frac {(2+4x)^{k}}{k}})$

Number 8

Radius of convergence of log(3+4x) about the point ${\widehat {x}}=-.5$

${\widehat {x}}=-.5$
$\sum _{n=0}^{\infty }(-1)^{n+1}{\frac {4^{n}}{n}}(x+.5)^{n}$
$R_{c}=lim_{n\rightarrow \infty }[{(-1)^{n+2}}{\frac {4^{n+1}}{n+1}}(.25)^{n+1}*{\frac {n}{4^{n}(-1)^{n+1}(.25)^{n}}}]^{-1}$
Cancelling some terms out, you get
$R_{c}=lim_{n\rightarrow \infty }{\frac {-(n+1)}{n}}$
Using L'Hopitals Rule, you get
$R_{c}=lim_{n\rightarrow \infty }{\frac {1}{1}}=1$

Number 9

Radius of convergence of log(3+4x) about the point ${\widehat {x}}=-.25$

${\widehat {x}}=-.25$

$\sum _{n=0}^{\infty }(-1)^{n+1}{\frac {4^{n}}{n}}(x+.25)^{n}$
$R_{c}=lim_{n\rightarrow \infty }[{(-1)^{n+2}}{\frac {4^{n+1}}{n+1}}(.0625)^{n+1}*{\frac {n}{4^{n}(-1)^{n+1}(.0625)^{n}}}]^{-1}$
Cancelling some terms out, you get
$R_{c}=lim_{n\rightarrow \infty }{\frac {-4(n+1)}{n}}$
Using L'Hopitals Rule, you get
$R_{c}=lim_{n\rightarrow \infty }{\frac {4}{1}}=4$

Number 10

Radius of convergence of log(3+4x) about the point ${\widehat {x}}=1$

${\widehat {x}}=1$
$\sum _{n=0}^{\infty }(-1)^{n+1}{\frac {4^{n}}{n}}(x-1)^{n}$
$R_{c}=lim_{n\rightarrow \infty }[{(-1)^{n+2}}{\frac {4^{n+1}}{n+1}}(1)^{n+1}*{\frac {n}{4^{n}(-1)^{n+1}(1)^{n}}}]^{-1}$
Cancelling some terms out, you get
$R_{c}=lim_{n\rightarrow \infty }{\frac {-(n+1)}{4n}}$
Using L'Hopitals Rule, you get
$R_{c}=lim_{n\rightarrow \infty }{\frac {1}{4}}=1/4$

Number 11

Radius of convergence of log(3+4x) about any given point ${\widehat {x}}$

$\sum _{n=0}^{\infty }(-1)^{n+1}{\frac {4^{n}}{n}}(x-{\widehat {x}})^{n}$
$R_{c}=lim_{n\rightarrow \infty }[{(-1)^{n+2}}{\frac {4^{n+1}}{n+1}}({\widehat {x}}^{2})^{n+1}*{\frac {n}{4^{n}(-1)^{n+1}(({\widehat {x}}^{2})^{n})}}]^{-1}$

Honor Pledge

On our honor, we solved this problem on our own, without aid from online solutions or solutions from previous semesters.

Problem 3:

Problem Statement

Use the Determinant of the Matrix of Components and the Gramian to verify the linear independence of the two vectors $b_{1}$ and $b_{2}$.

$\mathbf {b} _{1}=5\mathbf {e} _{1}-3\mathbf {e} _{2}$

$\mathbf {b} _{2}=-2\mathbf {e} _{1}+4\mathbf {e} _{2}$

Solution

Determinant of the Matrix of Components

The Matrix of components of the vectors $b_{1}$ and $b_{2}$ is

${\begin{vmatrix}5&-3\\-2&4\end{vmatrix}}=(5)(4)-(-3)(-2)=20-6=14\neq 0$

So the vectors $b_{1}$ and $b_{2}$ are linearly independent.

Gramian

For vectors, the Gramian is defined as:

$\ {\boldsymbol {\Gamma }}(b_{1},b_{2})={\begin{bmatrix}\langle b_{1},b_{1}\rangle &\langle b_{1},b_{2}\rangle \\\langle b_{2},b_{1}\rangle &\langle b_{2},b_{2}\rangle \end{bmatrix}}$

where:

$\ \langle b_{i},b_{j}\rangle =b_{i}\cdot b_{j}$

For the given vectors, the dot products are:

$\ \langle b_{1},b_{1}\rangle =(5e_{1}-3e_{2})\cdot (5e_{1}-3e_{2})=(5)(5)+(-3)(-3)=25+9=34$

$\ \langle b_{1},b_{2}\rangle =(5e_{1}-3e_{2})\cdot (-2e_{1}+4e_{2})=(5)(-2)+(-3)(4)=-10-12=-22$

$\ \langle b_{2},b_{1}\rangle =(-2e_{1}+4e_{2})\cdot (5e_{1}-3e_{2})=(-2)(5)+(4)(-3)=-10-12=-22$

$\ \langle b_{2},b_{2}\rangle =(-2e_{1}+4e_{2})\cdot (-2e_{1}+4e_{2})=(-2)(-2)+(4)(4)=4+16=20$

So the Gramian matrix becomes: $\ {\boldsymbol {\Gamma }}(b_{1},b_{2})={\begin{bmatrix}34&-22\\-22&20\end{bmatrix}}$

Finding the determinant of the Gramian matrix gives the Gramian:

$\ \Gamma =(34)(20)-(-22)(-22)=680-484=156\neq 0$

So the vectors $b_{1}$ and $b_{2}$ are linearly independent.

Honor Pledge

On our honor, we solved this problem on our own, without aid from online solutions or solutions from previous semesters.

Problem 4: Wronskian and Gramian

Problem Statement

Use both the Wronskian and the Gramain to find whether the following functions are linearly independent. Consider the domain of these functions to be [-1, +1] for the construction of the Gramian matrix.

$f(x)=x,g(x)=x^{5}$

$f(x)=cos2x,g(x)=sin4x$

Solution

Wronskian:

$W(f,g):={\begin{bmatrix}f&g\\f'&g'\end{bmatrix}}=fg'-gf'$

Function is linearly independent if $W(f,g)\neq 0$

1) $f(x)=x,g(x)=x^{5}$

$f(x)=x,f'(x)=1$
$g(x)=x^{5},g'(x)=5x^{4}$
$W(f,g):={\begin{bmatrix}x&x^{5}\\1&5x^{4}\end{bmatrix}}=5x^{5}-x^{5}=4x^{5}\neq 0$ so function is linearly independent.

2)$f(x)=cos2x,g(x)=sin4x$

$f(x)=cos2x,f'(x)=-2sin2x$
$g(x)=sin4x,g'(x)=4cos4x$

$W(f,g):={\begin{bmatrix}cos2x&sin4x\\-2sin2x&4cos4x\end{bmatrix}}=cos2x*4cos4x+sin4x*2sin2x\neq 0$ so function is linearly independent.

Gramian:
$\Gamma (f,g):={\begin{bmatrix}<f,f>&<f,g>\\<g,f>&<g,g>\end{bmatrix}}$

Function is linearly independent if $\Gamma (f,g)\neq 0$

1) $f(x)=x,g(x)=x^{5}$
$<f,f>=\int _{-1}^{1}x^{2}dx={\frac {2}{3}}$
$<f,g>=\int _{-1}^{1}x^{6}dx={\frac {2}{7}}$
$<g,f>=\int _{-1}^{1}x^{6}dx={\frac {2}{7}}$
$<g,g>=\int _{-1}^{1}x^{1}0dx={\frac {2}{11}}$

$\Gamma (f,g):={\begin{bmatrix}{\frac {2}{3}}&{\frac {2}{7}}\\{\frac {2}{7}}&{\frac {2}{11}}\end{bmatrix}}\neq 0$ so function is linearly independent.

2) $f(x)=cos2x,g(x)=sin4x$
$<f,f>=\int _{-1}^{1}cos2x*cos2xdx={\frac {1}{4}}(4+sin4)=.818$
$<f,g>=\int _{-1}^{1}cos2x*sin4xdx=[.5cos^{2}x-1/12cos6x]_{-}^{1}1=0$
$<g,f>=\int _{-1}^{1}cos2x*sin4xdx=0$
$<g,g>=\int _{-1}^{1}sin4x*sin4xdx=1+{\frac {sin8}{8}}=.876$

$\Gamma (f,g):={\begin{bmatrix}.818&0\\0&.876\end{bmatrix}}\neq 0$ so function is linearly independent.

Honor Pledge

On our honor, we solved this problem on our own, without aid from online solutions or solutions from previous semesters.