University of Florida/Egm4313/IEA-f13-team10/R3

Report 3

Problem 1

Problem Statement

Find the complete homogeneous solution using variation of parameters
$16y''+8y'+y=r(x)$

Solution

$16y''+8y'+y=0$

The solution is $y=e^{rx}$
Therefore, $y'=re^{rx}$ and $y''=r^{2}e^{rx}$

Plugging this back into the original homogeneous equation, $e^{rx}(16r^{2}+8r+1)=0$
$16r^{2}+8r+1=0$
$(4r+1)(4r+1)=0$ so $r=-1/4$
$y_{h}=y_{1,h}+y_{2,h}=y_{1,h}+xy_{1,h}$
$y_{h}(x)=cbar_{1}e^{-x/4}+cbar_{2}xe^{-x/4}$

Checking the answer
$y_{2}=u(x)y_{1}$
$y=e^{-x/4}$
$y=-1/4*e^{-x/4}$
$y=1/16*e^{-x/4}$
$y'_{2}=u'y_{1}+uy'_{1}$
$y''_{2}=u''y_{1}+2u'y'_{1}+uy''$

Plugging this into the original homogeneous equation
$16(u''y_{1}+2u'y'_{1}+uy''_{1})+8(u'y_{1}+uy'_{1})+(uy)=0$

Plugging in values for y and its derivatives, everything cancels out to zero.

Honor Pledge

On our honor, we solved this problem on our own, without aid from online solutions or solutions from previous semesters.

Problem 2

Problem Statement

Find and plot the solution for the L2-ODE_CC
$y''-10y'+25y=r(x)$ $y(0)=1,y'(0)=1,r(x)=0$

Solution

$y''-10y'+25y=r(x)$
$y''-10y'+25y=0$
This is a linear, first order ODE with constant coefficients.

To find the general solution to this ODE set $y=e^{rx}$

so that $y'=re^{rx}$ and $y''=r^{2}e^{rx}$

Substituting in y to the ODE and factoring out $e^{rx}$ we get:

$r^{2}-10r+25=0$

Using the quadratic formula to solve for r we get

$r={\frac {-b\pm {\sqrt {b^{2}-4ac}}}{2a}}$ where $a=1,b=-10,$ and $c=25$

Solving to get $r=5$

Since we have a repeated root, we need to find v(x) so that y2(x) = v(x)y1(x)

Taking the first and second derivative of y2(x) we get:

$y_{2}'(x)=v'(x)y_{1}(x)+v(x)y_{1}'(x)$
$y_{2}''(x)=v''(x)y_{1}(x)+2v'(x)y_{1}(x)+v(x)y_{1}''(x)$

Substituting into the original ODE, we get:

$(v''(x)y_{1}(x)+2v'(x)y_{1}(x)+v(x)y_{1}''(x))-10(v'(x)y_{1}(x)+v(x)y_{1}'(x))+25(v(x)y1(x))=0$
solving for $v''(x)=0$ so v(x) = kx + c

So y2(x) = x y1(x)

We get the general solution $y(x)=C_{1}e^{5x}+C_{2}xe^{5x}$

Now with the initial values y(0) = 1 and y'(0) = 0

$y(0)=C_{1}=1$

$y'(0)=5(1)+C_{2}=0$

$C_{1}=1$ , $C_{2}=-5$

$y(x)=e^{5x}-5xe^{5x}$

Honor Pledge

On our honor, we solved this problem on our own, without aid from online solutions or solutions from previous semesters.

Problem 3

Problem Statement

Problem Sec 2.4 problem 3

How does the frequency of the harmonic oscillation change if we (i) double the mass (ii) take a spring of twice the modulus?

Problem Sec 2.4 problem 4

Could you make a harmonic oscillation move faster by giving the body a greater push?

Solution

Problem Sec 2.4 problem 3

Part 1

$\omega ={\sqrt {\frac {k}{m}}}$
$f={\frac {\omega }{2\pi }}={\frac {\sqrt {\frac {k}{m}}}{2\pi }}$
Now double the mass
$f={\frac {\omega }{2\pi }}={\frac {\sqrt {\frac {k}{2m}}}{2\pi }}$
$f={\frac {\sqrt {\frac {k}{m}}}{2{\sqrt {2}}\pi }}$
The frequency is decreased by ${\sqrt {2}}$.

Part 2

Multiply k by 2
$f={\frac {{\sqrt {2}}{\sqrt {\frac {k}{m}}}}{2\pi }}$
The frequency is increased by ${\sqrt {2}}$.

Problem Sec 2.4 problem 4

No because frequency depends on the ratio of the spring modulus and mass.

Honor Pledge

On our honor, we solved this problem on our own, without aid from online solutions or solutions from previous semesters.

Problem 4

Problem Statement

Section 2.4 Problem 16

Show the maxima of an underdamped motion occur at equidistant t-values and find the distance.

Section 2.4 Problem 17

Determine the values of t corresponding to the maxima and minima of the oscillation $y(t)=e^{-t}sin(t)$. Check your result by graphing y(t).

Part 1=

The general solution of underdamped motion is
$y(t)=Ce^{\alpha t}cos(\omega ^{*}t-\delta )$
The maximas occur at $y(t)=Ce^{\alpha t}$
Set the two equations equal to each other a solve for t.
$Ce^{\alpha t}=Ce^{\alpha t}cos(\omega ^{*}t-\delta )$
$1=cos(\omega ^{*}t-\delta )$
$\cos ^{-1}(1)=\omega ^{*}t-\delta$
$t={\frac {2\pi n+\delta }{\omega ^{*}}}$ where n=0,1,2,3.....

Part 2

$tan(\delta )={\frac {B}{A}}$ shows delta is a constant.
The periodic distance between maximas is ${\frac {2\pi }{\omega }}$

Section 2.4 Problem 17

$y(t)=e^{-t}sin(t)$
$y'(t)=e^{-t}[-sin(t)+cos(t)]$

To find critical points, set y'(t)=0

$e^{-t}[-sin(t)+cos(t)]=0$
$[-sin(t)+cos(t)]=0$
$sin(t)=cos(t)$
${\frac {sin(t)}{cos(t)}}=1$
$tan(t)=1$
$t=\tan ^{-1}(1)$
$t=n\pi +{\frac {\pi }{4}}$where n=0,1,2,3...

As seen in the graph, the maximum of t was at ${\frac {\pi }{4}}$ and the minimum was at ${\frac {5\pi }{4}}$.

Honor Pledge

On our honor, we solved this problem on our own, without aid from online solutions or solutions from previous semesters.

Problem 5

Problem Statement

Using the formula for Taylor series at x = 0 (the origin, i.e., McLaurin series), develop into Taylor series at the origin x = 0 for the following functions: cos x, sin x, exp(x), tan x, and write these series in compact form with the summation sign and a single summand.

Solution

Part 1
cos x
Taylor Series:
$f(x)=sum({\frac {1}{n!}})(f^{(}n)(x_{o}))(x-x_{o})^{n})$
when $x_{o}=0$

$f(x)=\cos(x):$

$f(0)=\cos(0)=1$

$f'(0)=-\sin(0)=0$

$f''(0)=-\cos(0)=-1$

$f'''(0)=\sin(0)=0$

$f(0)=sum({\frac {(-1)^{n}x^{2}n}{2n!}})$

$f(0)=1-{\frac {x^{2}}{2!}}+{\frac {x^{4}}{4!}}-{\frac {x^{6}}{6!}}+{\frac {x^{8}}{8!}}-{\frac {x^{1}0}{10!}}+....$

n = 0, 1, 2, 3 ... N

2n = 0, 2, 4, 6, ... 2N

$cos(x)=sum({\frac {(-1)^{n}x^{2}n}{2n!}})$

Part 2
sin x
Taylor Series:
$f(x)=sum({\frac {1}{n!}})(f^{(}n)(x_{o}))(x-x_{o})^{n})$
when $x_{o}=0$

$f(x)=\sin(x):$

$f(0)=\sin(0)=0$

$f'(0)=\cos(0)=1$

$f''(0)=-\sin(0)=0$

$f'''(0)=-\cos(0)=-1$

$f(0)=(-1)^{(}n+1)sum({\frac {x^{(}2n-1)}{(2n-1)!}})$

$sin(x)={\frac {x}{1!}}-{\frac {x^{2}}{3!}}+{\frac {x^{5}}{5!}}-{\frac {x^{7}}{7!}}+....$

$sin(x)=(-1)^{(}n+1)sum({\frac {x^{(}2n-1)}{(2n-1)!}})$

Part 3
exp x
Taylor Series:
$f(x)=sum({\frac {1}{n!}})(f^{(}n)(x_{o}))(x-x_{o})^{n})$
when $x_{o}=0$

$f(x)=e^{x}:$

$f(0)=e^{0}=1$

$f'(0)=e^{0}=1$

$f''(0)=e^{0}=1$

$f'''(0)=e^{0}=1$

$e^{x}={\frac {x}{1!}}+{\frac {x^{2}}{2!}}+{\frac {x^{3}}{3!}}+{\frac {x^{4}}{4!}}+....$

$e^{x}=sum({\frac {x^{n}}{n!}})$

Honor Pledge

On our honor, we solved this problem on our own, without aid from online solutions or solutions from previous semesters.

Problem 6

Problem Statement

Part 1
Find and plot the solution for the L2-ODE-CC
$y''+4y'+13y=r(x)$
Initial conditions: y(0) = 1, y'(0) = 0
No excitation: r(x) = 0

Part 2
In another Fig., superpose 3 Figs.: (a) this Fig.,
(b) the Fig. in R2.6 P. 5-6, (c) the Fig. in R2.1 P. 3-7.

Solution

Part 1
$y''+4y'+13y=0$

The characteristic equation of the given ODE is:

$\lambda ^{2}+4\lambda +13=0$

Using the quadratic formula to solve for $\lambda$

$\lambda ={\frac {-b\pm {\sqrt {b^{2}-4ac}}}{2a}}$ where $a=1,b=4,c=13$

Solving to get $\lambda ={\frac {-4\pm 6i}{2}}$

$\lambda _{1}=-2+3i$, $\lambda _{2}=-2-3i$

Therefore, the general solution of the given ODE is $y=e^{-2x}[C_{1}\cos 3x+C_{2}\sin 3x]$

Now we solve for $C_{1}$, $C_{2}$ using the given initial conditions

We have $y(0)=1$

Substituting $x=0,y=1$ into $y=e^{-2x}[C_{1}\cos 3x+C_{2}\sin 3x]$,

We get,

$1=e^{-2(0)}[C_{1}\cos 3(0)+C_{2}\sin 3(0)]$

$1=C_{1}\cos 3(0)+C_{2}\sin 3(0)$

$1=C_{1}$

We have $y'(0)=0$

Differentiating $y=e^{-2x}[C_{1}\cos 3x+C_{2}\sin 3x]$, we get:

$y'=-2e^{-2x}[C_{1}\cos 3x+C_{2}\sin 3x]+e^{-2x}[-3C_{1}\cos 3x+3C_{2}\sin 3x]$
.

Substituting $x=0,y'=0$ into $y'=-2e^{-2x}[C_{1}\cos 3x+C_{2}\sin 3x]+e^{-2x}[-3C_{1}\cos 3x+3C_{2}\sin 3x]$, we get:

$0=-2e^{-2(0)}[C_{1}\cos 3(0)+C_{2}\sin 3(0)]+e^{-2(0)}[-3C_{1}\cos 3(0)+3C_{2}\sin 3(0)]$

$0=-2C_{1}+3C_{2}$

$2C_{1}=3C_{2}$

$2(1)=3C_{2}$

$C_{2}=2/3$

Therefore, we get $C_{1}=1,C_{2}=2/3$

Hence the solution of the given ODE is $y=e^{-2x}[\cos 3x+2/3\sin 3x]$

Fig. 1:

Part 2
$x=0:0.2:20$
$y=e^{-2x}[\cos 3x+2/3\sin 3x]$
$y_{2}=e^{5x}-5xe^{5x}$
$y_{3}={\frac {5}{7}}e^{-2x}+{\frac {2}{7}}e^{5x}$

Fig. 2:

Fig. 3:

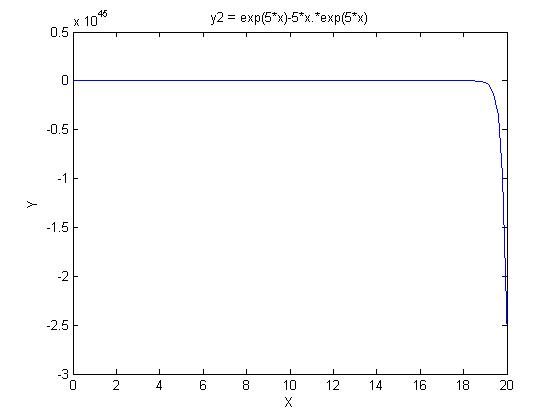
Fig. 4:

Honor Pledge

On our honor, we solved this problem on our own, without aid from online solutions or solutions from previous semesters.

Problem 7

Problem Statement

Consider the same system as in the Example p.7-3, i.e., the same L2-ODE-CC (4) p.5-5 and initial condi- (2) p.3-4, but with the following excitation: $r(x)=7e^{5x}-2x^{2}$

Solution

$y''-10y'+25y=7e6{5x}-2x^{2}$

$y_{p}=C_{1}x^{2}e^{5x}-C_{2}x^{3}$

Replacing $y$ with $y_{p}$ and after simplifying we get,

$2C_{1}e^{5x}-C_{2}x(25x^{2}-30x+6)=7e^{5x}-2x$

The root here is $\lambda =5$. So we can solve for our constants,

$C_{1}={\frac {7}{2}}$
$C_{2}={\frac {2}{481}}$

$y(x)=y_{h}+y_{p}=c_{1}e^{5x}+c_{2}xe^{5x}+{\frac {7}{2}}x^{2}e^{5x}-{\frac {2}{481}}x^{3}$

Using the initial conditions y(0)=4 and y'(0)=-5 we can solve for our constants,

$c_{1}=4$
$c_{2}=-1$

So the solution is,

$y=4e^{5x}+xe^{5x}+{\frac {7}{2}}x^{2}e^{5x}-{\frac {2}{481}}x^{3}$

Honor Pledge

On our honor, we solved this problem on our own, without aid from online solutions or solutions from previous semesters.

Problem 8

Problem Statement

Plot the error between the exact derivative and the approximate derivative, i.e.

$xe^{\lambda x}-{\frac {e^{(\lambda +\epsilon )x}-e^{\lambda x}}{\epsilon }}$ from

$-15\leq x\leq 15$

For ε = .0001, .0003, .0006, and .001 and λ =.3

Solution

Since the above equation is the error between the exact derivative and the approximate derivative, it must be plotted with the correct values of \epsilon and \lambda, from x = -15 to 15

Case 1

ε=.0001

Case 2

ε=.0003

Case 3

ε=.0006

Case 4

ε=.001

Honor Pledge

On our honor, we solved this problem on our own, without aid from online solutions or solutions from previous semesters.

Problem 9

Problem Statement

Find the complete solution for $y''-3y'+2y=4x^{2}$, with the initial conditions
$y(0)=1$, $y'(0)=0$
plot the solution y(x)

Solution

Particular Solution
$y_{p}(x)=Ax^{2}+Bx+c$
$y_{p}'=2Ax+B$
$y_{p}''=2A$
$2A-6Ax-3B+2Ax^{2}+2Bx+2c=4x^{2}$
$2A=4$

$-6A+2B=0$

$2A-3B+2C=0$


$A=2$
$B=6$
$C=7$


$y_{p}(x)=2x^{2}+6x+7$


Homogeneous Solution
$y_{h}(x)=c_{1}e^{x}+c_{2}e^{(}2x)$
$y_{h}'=c_{1}e^{x}+2c_{2}e^{(}2x)$


Initial conditions
$y(0)=1$
$1=c_{1}+c_{2}$

$y'(0)=0$
$0=c_{1}+2c_{2}$


$c_{1}=2,c_{2}=-1$


General Solution
$y_{g}=2e^{x}-e^{(}2x)+2x^{x}+6x+7$

Honor Pledge

On our honor, we solved this problem on our own, without aid from online solutions or solutions from previous semesters.