Real modulus/Introduction/Section

Definition

For a real number ${}x\in \mathbb {R}$, the modulus is defined in the following way.

${}\vert {x}\vert ={\begin{cases}x\,,{\text{ if }}x\geq 0\,,\\-x,\,{\text{ if }}x<0\,.\end{cases}}\,$

So the modulus (also called the absolute value) is never negative and has only at ${}x=0$ the value ${}0$, elsewhere it is always positive. The mapping

$\mathbb {R} \longrightarrow \mathbb {R} ,x\longmapsto \vert {x}\vert ,$

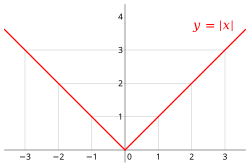
is called the modulus function. Its graph consists of two half lines; such a function is called piecewisely linear.

Lemma

The modulus function

$\mathbb {R} \longrightarrow \mathbb {R} ,x\longmapsto \vert {x}\vert ,$

fulfills the following properties (${}x,y$ are arbitrary real numbers).

1. ${}\vert {x}\vert \geq 0$.
2. ${}\vert {x}\vert =0$ if and only if ${}x=0$.
3. ${}\vert {x}\vert =\vert {y}\vert$ if and only if ${}x=y$ or ${}x=-y$ holds.
4. ${}\vert {y-x}\vert =\vert {x-y}\vert$.
5. ${}\vert {xy}\vert =\vert {x}\vert \vert {y}\vert$.
6. For ${}x\neq 0$ we have ${}\vert {x^{-1}}\vert =\vert {x}\vert ^{-1}$.
7. We have ${}\vert {x+y}\vert \leq \vert {x}\vert +\vert {y}\vert$ (triangle inequality for the modulus).
8. ${}\vert {x+y}\vert \geq \vert {x}\vert -\vert {y}\vert$.

Proof

See exercise.

$\Box$