Rational numbers
Appearance
(Redirected from Rational number)
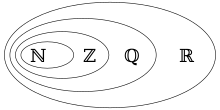
A rational number is a number that can be expressed as the quotient or fraction p/q of two integers, a numerator p and a non-zero denominator q. The set of all rational numbers, also referred to as "the rationals", the field of rationals, or the field of rational numbers is usually denoted by a boldface Q (or blackboard bold , Unicode U+1D410 𝐐 MATHEMATICAL BOLD CAPITAL Q, or U+211A ℚ DOUBLE-STRUCK CAPITAL Q).[1]




