Quantum mechanics/Wave equations in quantum mechanics
This collection of thoughts about quantum mechanics is based on the belief[1] that quantum mechanics can be introduced in a non-mathematical but rigorous way. Central to that belief is the fact that there is nothing mysterious about a wave equation. For that reason the first image in the gallery shows how simple Newtonian mechanics can be used to introduce a simple wave.
Gallery
[edit | edit source]-
1. transverse string waves
-
2. refraction of light
-
3. Eikonal approximation
-
4. wave packet
-
5. Klein Gordon equation
-
6. particle in two places
- Newtonian physics can analyze waves if matter can be broken up into cells that move according to F=ma.. To model a violin string, we need to imagine an infinite number of these masses connected by springs, something that requires mathematical complexity beyond the scope of this essay.
- Waves are known to bend, such as in this mirage (click here). for an informative depiction of a mirage). To "bend" or change direction while in motion is to accelerate. This bending involves a two or three dimensional picture of a wave. In contrast, the string of lumped masses and springs in the previous image was one-dimensional.
- The Eikonal approximation is used to study light waves when the wavelength is short. In this image a wave is depicted that accelerates due to a gentle inhomogeniety in the medium that supports the wave. A good example of such a inhomogeniety occurs if you hold one end of a slinky from a height. The weight of the slinky causes more tension at the top than at the bottom. Homogeneity is important in physics because it greatly simplifies the mathematics.
- A wave packet is a pulse that is typically many wavelengths long. Beginning in 1905 there was growing confusion about the distinction between waves and particles. Light is obviously a wave that sometimes acts as a particle. Electrons were shown to exhibit wavelike properties. By 1926 this paradoxical behavior was largely understood.[2]
- It is important to understand that the lumped-sum wave model of figure 1 is very simple. If one makes simplifying assumptions about the spring, the equations have important properties, the most important being linearity. The figure shows a modification that preserves linearity and therefore is extremely easy to solve. Extra springs are attached perpendicular to the axis along which the wave propagates. Also each mass is drilled with two holes so that the mass may move only in the y direction. It is assumed that there is no friction. This models the famous Klein-Gordon equation of a relativistic particle in free space (i.e., with no forces acting on it).
- This last image summarizes the w:Copenhagen interpretation interpretation of quantum mechanics. Although it is possible to construct wave packets that move as particles, these wavepackets do not always behave as particles. This occurs in a number of ways. In this image, the wave strikes a sharp inhomogeniety, such as happens when light strikes glass. Part of the wave is transmitted and part is reflected. How can this happen? How can a particle go in two directions or be in two places at the same time? The question you should ask is what property of a particle can exist in two different places. The answer is probability. Quantum mechanics does tell where the electron is. It tells us where the eletron might be.
Why amplitude squared?
[edit | edit source]Probability is a real number. But the waves of quantum mechanics have solutions with real and imaginary parts (complex numbers). The product of a complex number and its complex conjugate is real and positive.[3]
It is customary to denote the wave amplitude by the Greek letter psi, Ψ. We know that is real, so let us see if we can connect this to probability.
When speaking of the probability of a continuous variable like position (x), we must use the concept of probability density. Consider the small increment dx (or Δx). The larger the value of the larger the probability of being in that increment. It is 7 times more likely that this is the week of your birthday than that today is your birthday.
- Therefore probability of a particle being with an interval dx is directly proportional to dx.
Question: Each of the following is a candidate for the probability of being with dx of x:
How do we know that the first is probability density?
Because only the first has the necessary property that the sum (integral) over all probabilities never changes. You can verify this by looking at both reflected and transmitted pulses and convincing yourself that the net area seems to stay constant:
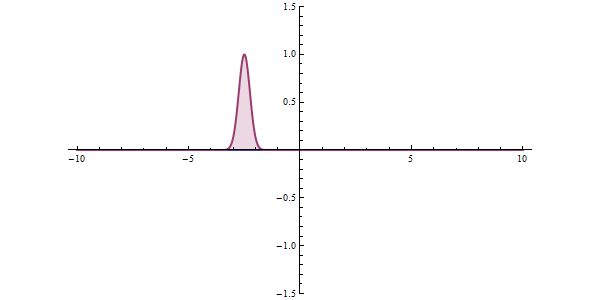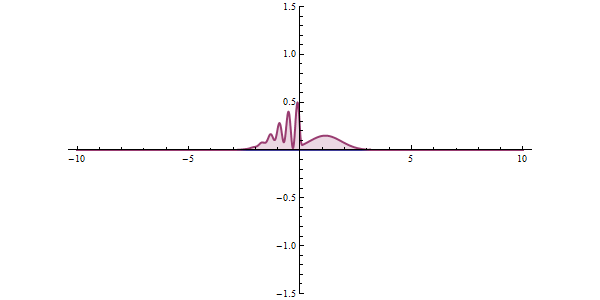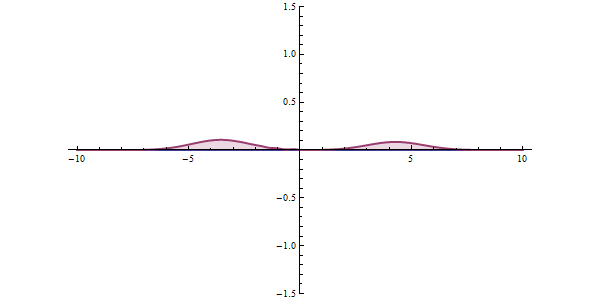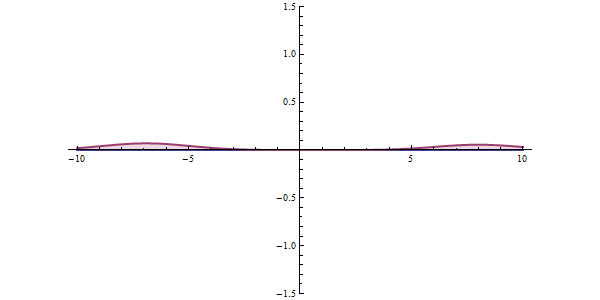
References and footnotes
[edit | edit source]Wikivercity is still very much the small town library with very few books. But things are happening on this topic. From least advanced to most advanced:
- How things work college course/Quantum mechanics timeline
- Topic:Advanced Classical Mechanics/The Eikonal Approximation and Classical Particle Motion
- Advanced_Classical_Mechanics/Continuum_Mechanics
<\references>
- ↑ "Belief" is an odd word, but in the field of science pedagogy we know very little.
- ↑ See w:Copenhagen interpretation
- ↑ Positive is good because probability also has to be positive.






