Materials Science and Engineering/Doctoral review questions/Daily Discussion Topics/01072008

The Kelvin-Stokes Theorem

An illustration of Kelvin-Stokes theorem with surface $\Sigma ,$ its boundary $\partial \Sigma ,$ and orientation $n.$

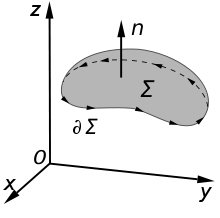
The classical Kelvin-Stokes theorem:

$\int _{\Sigma }\nabla \times \mathbf {F} \cdot d\mathbf {\Sigma } =\oint _{\partial \Sigma }\mathbf {F} \cdot d\mathbf {r} ,$

which relates the surface integral of the curl of a vector field over a surface $\Sigma$ in Euclidean three-space to the line integral of the vector field over its boundary, is a special case of the general Stokes theorem (with n = 2) once we identify a vector field with a 1 form using the metric on Euclidean three-space. The curve of the line integral ($\partial \Sigma$) must have positive orientation, such that $d\mathbf {r}$ points counterclockwise when the surface normal ($d\mathbf {\Sigma }$) points toward the viewer, following the right-hand rule.

Divergence Theorem

Mathematical Statement

A region V bounded by the surface S=∂V with the surface normal n.

Suppose V is a subset of Rⁿ (in the case of n = 3, V represents a volume in 3D space) which is compact and has a piecewise smooth boundary. If F is a continuously differentiable vector field defined on a neighborhood of V, then we have

$\iiint \limits _{V}\left(\nabla \cdot \mathbf {F} \right)dV=\iint \limits _{\partial V}\mathbf {F} \cdot \mathbf {n} \,dS.$

The left side is a volume integral over the volume V, the right side is the surface integral over the boundary of the volume V. Here ∂V is quite generally the boundary of V oriented by outward-pointing normals, and n is the outward pointing unit normal field of the boundary ∂V. (dS may be used as a shorthand for ndS.) In terms of the intuitive description above, the left-hand side of the equation represents the total of the sources in the volume V, and the right-hand side represents the total flow across the boundary ∂V.

Example

A vector field on a sphere (this is not the field in the example).

Suppose we wish to evaluate

$\iint \limits _{S}\mathbf {F} \cdot \mathbf {n} \,dS,$

where S is the unit sphere defined by $x^{2}+y^{2}+z^{2}=1$ and F is the vector field

$\mathbf {F} =2x\mathbf {i} +y^{2}\mathbf {j} +z^{2}\mathbf {k} .$

The direct computation of this integral is quite difficult, but we can simplify it using the divergence theorem:

${\begin{aligned}\iint \limits _{S}\mathbf {F} \cdot \mathbf {n} \,dS&=\iiint \limits _{W}\left(\nabla \cdot \mathbf {F} \right)\,dV\\&=2\iiint \limits _{W}\left(1+y+z\right)\,dV\\&=2\iiint \limits _{W}\,dV+2\iiint \limits _{W}y\,dV+2\iiint \limits _{W}z\,dV.\end{aligned}}$

Since the functions $y$ and $z$ are odd on $S$ (which is a symmetric set in respect to the coordinate planes), one has

$\iiint \limits _{W}y\,dV=\iiint \limits _{W}z\,dV=0.$

Therefore,

$2\iiint \limits _{W}\left(1+y+z\right)dV=2\iiint \limits _{W}\,dV={\frac {8\pi }{3}}$

because the unit ball W has volume 4π/3.

Original Ampère's circuital law

An electric current produces a magnetic field.

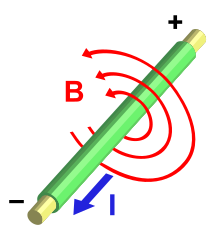
In its historically original form, Ampère's Circuital law relates the magnetic field $\mathbf {B}$ to its source, the current density $\mathbf {J}$. The equation is not in general correct (see "Maxwell's correction" below), but is correct in the special case where the electric field is constant (unchanging) in time.

The law can be written in two forms, the "integral form" and the "differential form". The forms are equivalent, and related by the Kelvin-Stokes theorem.

Integral form

In SI units, (the version in cgs units is in a later section), the "integral form" of the original Ampère's Circuital law is:

$\oint _{C}\mathbf {B} \cdot \mathrm {d} \mathbf {l} =\mu _{0}\iint _{S}\mathbf {J} \cdot \mathrm {d} \mathbf {S}$

or equivalently,

$\oint _{C}\mathbf {B} \cdot \mathrm {d} \mathbf {l} =\mu _{0}I_{\mathrm {enc} }$

where:

• $\oint _{C}$ is the closed line integral around contour (closed curve) C.
• $\mathbf {B}$ is the magnetic field in teslas.
• $\cdot$ is the vector dot product.
• $\mathrm {d} \mathbf {l}$ is an infinitesimal element (differential) of the contour C (i.e. a vector with magnitude equal to the length of the infinitesimal surface element, and direction equal to the direction of integration, see below),
• $\iint _{S}$ denotes an integral over the surface S enclosed by contour C (see below). The double integral sign is meant simply to denote that the integral is two-dimensional in nature.
• $\mu _{0}\!\$ is the magnetic constant.
• $\mathbf {J}$ is the current density (in amperes per square meter), both bound and free, through the surface S enclosed by contour C
• $\mathrm {d} \mathbf {S} \!\$ is the vector area of an infinitesimal element of surface S (i.e. a vector with magnitude equal to the area of the infinitesimal surface element, and direction normal to surface S),
• $I_{\mathrm {enc} }\!\$ is the net current that penetrates through the surface S, both bound and free.

Biot-Savart Law

The Biot-Savart law is used to compute the magnetic field generated by a steady current, i.e. a continual flow of charges, for example through a wire, which is constant in time and in which charge is neither building up nor depleting at any point. The equation is as follows:

$d\mathbf {B} ={\frac {\mu _{0}}{4\pi }}{\frac {Id\mathbf {l} \times \mathbf {r} }{r^{3}}}$

(in SI units), where

$\scriptstyle {I}$ is the current,

$\scriptstyle {d\mathbf {l} }$ is a vector, whose magnitude is the length of the differential element of the wire, and whose direction is the direction of conventional current,

$\scriptstyle {d\mathbf {B} }$ is the differential contribution to the magnetic field resulting from this differential element of wire,

$\scriptstyle {\mu _{0}}$ is the magnetic constant,

$\scriptstyle {\mathbf {r} }$ is the displacement vector from the wire element to the point at which the field is being computed,

$\scriptstyle {r}$ is the distance from the wire element to the point at which the field is being computed, and

$\scriptstyle {\times }$ denotes cross product.

To apply the equation, you choose a point in space at which you want to compute the magnetic field. Holding that point fixed, you integrate over the path of the current(s) to find the total magnetic field at that point. The application of this law implicitly relies on the superposition principle for magnetic fields, i.e. the fact that the magnetic field is a vector sum of the field created by each infinitesimal section of the wire individually.

Magnetic Flux

Magnetic flux, represented by the Greek letter Φ (phi), is a measure of quantity of magnetism, taking into account the strength and the extent of a magnetic field. The wiki:SI unit of magnetic flux is the weber (in derived units: volt-seconds), and the unit of magnetic field is the weber per square meter, or tesla.

Description

The flux through an element of area perpendicular to the direction of magnetic field is given by the product of the magnetic field and the area element. More generally, magnetic flux is defined by a scalar product of the magnetic field and the area element vector. Gauss's law for magnetism, which is one of the four Maxwell's equations, states that the total magnetic flux through a closed surface is zero. This law is a consequence of the empirical observation that magnetic monopoles do not exist or are not measurable.

The magnetic flux is defined as the integral of the magnetic field over an area:

$\Phi _{m}=\int \!\!\!\int \mathbf {B} \cdot d\mathbf {S} \,$

where

$\Phi _{m}\$ is the magnetic flux

B is the magnetic field

S is the surface (area).

We know from Gauss's law for magnetism that

$\nabla \cdot \mathbf {B} =0.\,$

The volume integral of this equation, in combination with the divergence theorem, provides the following result:

$\int \!\!\!\int \!\!\!\int _{V}\nabla \cdot \mathbf {B} \,d\tau =\oint \!\!\!\oint _{\partial V}\mathbf {B} \cdot d\mathbf {S} =0.$

In other words, the magnetic flux through any closed surface must be zero; there are no "magnetic charges".

By way of contrast, Gauss's law for electric fields, another of Maxwell's equations, is

$\nabla \cdot \mathbf {E} ={\rho \over \epsilon _{0}},$

where

E is the electric field,

$\rho$ is the free electric charge density, (not including dipole charges bound in a material),

$\epsilon _{0}$ is the permittivity of free space.

Note that this indicates the presence of electric monopoles, that is, free positive or negative charges.

The direction of the magnetic field vector $\mathbf {B}$ is by definition from the south to the north pole of a magnet (within the magnet). Outside of the magnet, the field lines will go from north to south.

A change of magnetic flux through a loop of conductive wire will cause an electromotive force, and therefore an electric current, in the loop. The relationship is given by Faraday's law:

${\mathcal {E}}=\oint \mathbf {E} \cdot d\mathbf {s} =-{d\Phi _{m} \over dt}.$

This is the principle behind an electrical generator.

Skin Effect

The skin effect is the tendency of an alternating electric current (AC) to distribute itself within a conductor so that the current density near the surface of the conductor is greater than that at its core. That is, the electric current tends to flow at the "skin" of the conductor. The skin effect causes the effective resistance of the conductor to increase with the frequency of the current. Skin effect is due to eddy currents set up by the AC current.

Skin Depth

When an electromagnetic wave interacts with a conductive material, mobile charges within the material are made to oscillate back and forth with the same frequency as the impinging fields. The movement of these charges, usually electrons, constitutes an alternating electric current, the magnitude of which is greatest at the conductor's surface. The decline in current density versus depth is known as the skin effect and the skin depth is a measure of the distance over which the current falls to 1/e of its original value. A gradual change in phase accompanies the change in magnitude, so that, at a given time and at appropriate depths, the current can be flowing in the opposite direction to that at the surface.

The skin depth is a property of the material that varies with the frequency of the applied wave. It can be calculated from the relative wiki:permittivity and conductivity of the material and frequency of the wave. First, find the material's complex permittivity, $\varepsilon _{c}$

$\varepsilon _{c}={{\varepsilon }\left(1-j{{\sigma } \over {\omega \varepsilon }}\right)}\qquad \qquad (1)$

where:

$\varepsilon$ = permittivity of the material of propagation

$\omega$ = angular frequency of the wave

$\sigma$ = electrical conductivity of the material of propagation

$j$ = the imaginary unit

Thus, the propagation constant, $k$, will also be a complex number, and can be separated into real and imaginary parts.

$k_{c}={\omega }{\sqrt {\mu \varepsilon _{c}}}=\alpha +j\beta$ = $j\omega {\sqrt {\mu \varepsilon \left({1-{\frac {j\sigma }{\omega \varepsilon }}}\right)}}\qquad \qquad (2)$

The constants can also be expressed as

$\alpha ={\omega }{\sqrt {{{\mu \varepsilon } \over 2}\left({\sqrt {1+\left({{\sigma } \over {\omega \epsilon }}\right)^{2}}}-1\right)}}\qquad \qquad (3)$

$\beta ={\omega }{\sqrt {{{\mu \varepsilon } \over 2}\left({\sqrt {1+\left({{\sigma } \over {\omega \epsilon }}\right)^{2}}}+1\right)}}\qquad \qquad (4)$

where:

$\mu$ = permeability of the material

$\alpha$ = attenuation constant of the propagating wave

The solution of the equation above is if it represent a uniform wave propagating in the +z-direction

$E_{x}=E_{0}e^{-\alpha z}e^{-j\beta z}\qquad \qquad (5)$

The first term in the solution decreases as z increases and is for this reason an attenuation term where $\alpha$ is an attenuation constant with the unit Np/m (Neper). If $\alpha =1$ then a unit wave amplitude decreases to a magnitude of $e^{-1}$ Np/m.

It can be seen that the imaginary part of the complex permittivity increases with frequency, implying that the attenuation constant also increases with frequency. Therefore, a high frequency wave will only flow through a very small region of the conductor (much smaller than in the case of a lower frequency current), and will therefore encounter more electrical resistance (due to the decreased surface area).

A good conductor is per definition if $1\ll \sigma /\varepsilon \omega$ why we can neglect 1 in equation (2) and it turns to

$k_{c}={\sqrt {j}}\,{\sqrt {\mu \omega \sigma }}={\frac {1+j}{\sqrt {2}}}{\sqrt {\mu 2\pi f\sigma }}=(1+j){\sqrt {\pi f\mu \sigma }}\qquad \qquad (6)$

The skin depth is defined as the distance $\delta$ through which the amplitude of a traveling plane wave decreases by a factor $e^{-1}$ and is therefore

$\delta ={\frac {1}{\alpha }}\qquad \qquad (7)$

and for a good conductor is it defined as

$\delta ={\frac {1}{\sqrt {\pi f\mu \sigma }}}\qquad \qquad (8)$

The term "skin depth" traditionally assumes ω real. This is not necessarily the case; the imaginary part of ω characterizes' the waves attenuation in time. This would make the above definitions for α and β complex, and so they would need to be redefined so that $Im\{k_{c}\}=\beta$.

The same equations also apply to a lossy dielectric. Defining

$\varepsilon _{c}={\left({\varepsilon '}-j{\varepsilon ''}\right)}$

replace $\varepsilon$ with $\varepsilon '$, and ${\sigma \over {\omega \varepsilon }}$with $\varepsilon '' \over {\varepsilon '}$

Rayleigh Scattering

Rayleigh scattering (named after Lord Rayleigh) is the scattering of light or other electromagnetic radiation by particles much smaller than the wavelength of the light. It can occur when light travels in transparent solids and liquids, but is most prominently seen in gases. Rayleigh scattering of sunlight in clear atmosphere is the main reason why the sky is blue. Rayleigh and cloud-mediated scattering contribute to diffuse light (direct light being sunrays).

For scattering by particles similar to or larger than a wavelength, see Mie theory or discrete dipole approximation (they apply to the Rayleigh regime as well).

Small Size Parameter Approximation

The size of a scattering particle is defined by the ratio (x) of its characteristic dimension (r) and wavelength (λ):

$x={\frac {2\pi r}{\lambda }}$.

Rayleigh scattering can be defined as scattering in small size parameter regime x < < 1. The amount of Rayleigh scattering that occurs to a beam of light is dependent upon the size of the particles and the wavelength of the light; in particular, the scattering coefficient, and hence the intensity of the scattered light, varies for small size parameter inversely with the fourth power of the wavelength. Scattering from larger spherical particles is explained by the Mie theory for arbitrary size parameter x including small size parameter - in this case Mie theory reduces to Rayleigh approximation.

The intensity I of light scattered by a single small particle from a beam of unpolarized light of wavelength λ and intensity I0 is given by:

$I=I_{0}{\frac {1+\cos ^{2}\theta }{2R^{2}}}\left({\frac {2\pi }{\lambda }}\right)^{4}\left({\frac {n^{2}-1}{n^{2}+2}}\right)^{2}\left({\frac {d}{2}}\right)^{6},$

where R is the distance to the particle, θ is the scattering angle, n is the refractive index of the particle, and d is the diameter of the particle.

The angular distribution of Rayleigh scattering, governed by the 1+cos² θ) term, is symmetric in the plane normal to the incident direction of the light, and so the forward scatter equals the backwards scatter. Integrating over the sphere surrounding the particle gives the Rayleigh scattering cross section σ_s:

$\sigma _{s}={\frac {2\pi ^{5}}{3}}{\frac {d^{6}}{\lambda ^{4}}}\left({\frac {n^{2}-1}{n^{2}+2}}\right)^{2}.$

The Rayleigh scattering coefficient for a group of scattering particles is the number of particles per unit volume N times the cross-section. As with all wave effects, in incoherent scattering the scattered powers add arithmetically, while in coherent scattering, such as if the particles are very near each-other, the fields add arithmetically and the sum must be squared to obtain the total scattered power.

The strong wavelength dependence of the scattering ((~λ^-4)) means that blue light is scattered much more than red light. In the atmosphere, this results in blue wavelength being scattered to a greater extent than longer wavelengths, and so one sees blue light coming from all regions of the sky. Direct radiation (from definition) is coming directly from the Sun. Rayleigh scattering is a good approximation to the manner in which light scattering occurs within various media for which scattering particles have small size parameter.

Mie Theory

Mie theory, also called Lorenz-Mie theory or Lorenz-Mie-Debye theory, is a complete analytical solution of Maxwell's equations for the scattering of electromagnetic radiation by spherical particles (also called Mie scattering). Mie solution is named after its developer German physicist Gustav Mie. However, Danish physicist Ludvig Lorenz and others independently developed the theory of electromagnetic plane wave scattering by a dielectric sphere.

The term "Mie theory" is misleading, since it does not refer to an independent physical theory or law. The phrase "the Mie solution (to Maxwell's equations)" is therefore preferable. Currently, the term "Mie solution" is also used in broader contexts, for example when discussing solutions of Maxwell's equations for scattering by stratified spheres or by infinite cylinders, or generally when dealing with scattering problems solved using the exact Maxwell equations in cases where one can write separate equations for the radial and angular dependence of solutions.

In contrast to Rayleigh scattering, the Mie solution to the scattering problem is valid for all possible ratios of diameter to wavelength, although the technique results in numerical summation of infinite sums. In its original formulation it assumed an homogeneous, isotropic and optically linear material irradiated by an infinitely extending plane wave. However, solutions for layered spheres are also possible.

Mie theory is very important in meteorological optics, where diameter-to-wavelength ratios of the order of unity and larger are characteristic of many problems regarding haze and cloud scattering. A further application is in the characterization of particles via optical scattering measurements. The Mie solution is also important for understanding the appearance of common materials like milk, biological tissue and latex paint.

Dispersivity of Electrons and Electromagnetic Waves in Vacuum

Properties of Electromagnetic Radiation

Electromagnetic waves can be imagined as a self-propagating transverse oscillating wave of electric and magnetic fields. This diagram shows a plane linearly polarised wave propagating from left to right.

Electric and magnetic fields obey the properties of superposition, so fields due to particular particles or time-varying electric or magnetic fields contribute to the fields due to other causes. (As these fields are vector fields, all magnetic and electric field vectors add together according to vector addition.) These properties cause various phenomena including refraction and diffraction. For instance, a travelling EM wave incident on an atomic structure induces oscillation in the atoms, thereby causing them to emit their own EM waves. These emissions then alter the impinging wave through interference.

Since light is an oscillation, it is not affected by travelling through static electric or magnetic fields in a linear medium such as a vacuum. In nonlinear media such as some crystals, however, interactions can occur between light and static electric and magnetic fields - these interactions include the Faraday effect and the Kerr effect.

In refraction, a wave crossing from one medium to another of different density alters its speed and direction upon entering the new medium. The ratio of the refractive indices of the media determines the degree of refraction, and is summarized by Snell's law. Light disperses into a visible spectrum as light is shone through a prism because of refraction.