Electric Circuit Analysis/Resistors in Parallel

Wikiversity Electrical Engineering School
The Lessons in
ELECTRIC CIRCUITS ANALYSIS COURSE

Lesson 3: Review

What you need to remember from Resistors in Series.

If you ever feel lost, do not shy away from going back to the previous lesson & going through it again. You can learn by repetition.

Total Series Resistance: ( $R_{E}=R_{1}+R_{2}\!$ )

The total Resistance of resistors in series is the sum of the resistance of every resistor in series.

Voltage Divider Equation 2.3: $V_{1}={\frac {R_{1}\times V_{s}}{R_{1}+R_{2}}}$

Current through Resistors connected in Series is the same for all resistors.

Lesson 4: Preview

This Lesson is about Resistors in Parallel. The student/user is expected to understand the following at the end of the lesson.

Two resistors connected in Parallel: $R_{eq}={\frac {R_{1}\times R_{2}}{R_{1}+R_{2}}}$

Current Divider Principle: $I_{i}=I_{s}\times {\frac {R_{eq}}{R_{i}}}$

Lessons in Electric Circuit Analysis

Lesson #1:

Passive Sign Convention

Lesson #2:

Simple Resistive Circuits

Lesson #3:

Resistors in Series

Lesson #4:

Resistors in Parallel← You are here

Quiz Test:

Circuit Analysis Quiz 1

Lesson #5:

Kirchhoff's Voltage Law

Lesson #6:

Kirchhoff's Current Law

Lesson #7:

Nodal Analysis

Lesson #8:

Mesh Analysis

Quiz Test:

Circuit Analysis Quiz 2

Home Laboratory:

Circuit Analysis - Lab1

Part 1

Introduction

The best way to understand Parallel circuits is to start with the definition. A circuit is parallel to another circuit or several circuits if and only if they share common terminals; i.e. if both the branches touch each other's endpoints. Here is an example:

Figure 4.1: A Parallel circuit

R1, R2, and the voltage source are all in parallel. To prove this fact consider the top and bottom parts of the circuit.

Figure 4.2: Components in parallel share a common nodes

The areas in yellow are all connected together, as well as the areas in blue. So all the branches have the same terminals, which means that R1, R2, and the source are all in parallel.

If we take this discussion of the water flow analogy. Electric current can be seen as water and the conductors as water pipes.

Something interesting happens as the current reaches the common node of resistors that are connected in parallel: the total current is divided into the parallel branches.

Part 2

Voltage Rule

If two or more branches are parallel then the voltage across them is equal. So based on this we can conclude that $V_{R1}=V_{R2}=5\ V$ . However, unlike series resistors, the current across the branches is not necessarily equal.

Equivalent resistance

For series resistors to find the total resistance we simply add them together. For parallel resistors, it's a little more complicated. Instead, we use the following equation:

R_{eq}={\frac {1}{{\frac {1}{R_{1}}}+{\frac {1}{R_{2}}}+...+{\frac {1}{R_{n}}}}}

However, for the case of only two resistors, we can use the following simplified form:

Equation 4.2: Total Parallel Resistance

R_{eq}={\frac {R_{1}R_{2}}{R_{1}+R_{2}}}

It is well to note at this point that the total resistance of parallel-connected resistors will always be less than the resistance of the smallest of the individual resistors.

Current Rule

In series connection, we deduced that voltage is divided amongst resistors. For parallel-connected resistors, however, the current is divided. So, as we did with the voltage division principle, here is the mathematical formula:

Equation 4.3: Current Divider Formula

$I_{i}=I_{s}\times {\frac {R_{eq}}{R_{i}}}$

Using this formula you can work out the currents flowing through individual resistors.

Part 3

Application

We have spent three lectures hacking on about what and why resistors and resistive circuits in two connection schemes are used (i.e. series and parallel connections). The question now is, where and how in real life do these connections happen?

One simple application of these connection schemes is the Shunt Application. In the electric measurement industry, most often enough, we wish to measure currents and voltages of very high magnitudes (in the range of 500kV upwards). The problem is that metering devices have delicate electronic components and usually have small voltage and current ratings.

The solution to the above problem is to have a metering device connected in parallel to a resistor, with the resistor thus called a "shunt" resistor since it is there to protect (shunt) the metering device as shown in Part 4.

Part 4

Figure 4.3: Application of Parallel Resistive circuits. Shunt connection

If we know the current rating of a device and the total current in the system, we can then work out the shunt current and, thus, the shunt resistance.

Part 5: Examples

Figure 4.4: Example 3.1

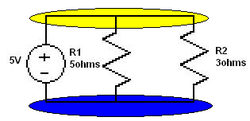
Figure 3.4 shows a parallel resistive circuit with the following parameters.
$V_{s}=10\ {\text{Volts}}$ ; $R_{1}=3\Omega$ ; $R_{2}=7\Omega$ .

Find $R_{eq}$ , ${\textstyle I_{1}}$ and ${\textstyle I_{2}}$

Solution: from Equation 4.2 we see that.

R_{eq}={\frac {R_{1}R_{2}}{R_{1}+R_{2}}}

Here are the solutions to the above problem:
${\begin{matrix}\ R_{eq}&=&{\frac {R_{1}\times R_{2}}{R_{1}+R_{2}}}\\\ \\\ &=&{\frac {(3\Omega \times 7\Omega )}{(3\Omega +7\Omega )}}\\\ \\\ &=&{\frac {21}{10}}\Omega \\\ \\\ &=&2.1\Omega \end{matrix}}$ .	${\begin{matrix}\ I_{1}&=&{\frac {V_{s}}{R_{1}}}\\\ \\\ &=&{\frac {10V}{3\Omega }}\\\ \\\ &=&3.33A\end{matrix}}$ .	${\begin{matrix}\ I_{2}&=&{\frac {V_{s}}{R_{2}}}\\\ \\\ &=&{\frac {10V}{7\Omega }}\\\ \\\ &=&1.43A\end{matrix}}$ .

Thus it can be said that the supply current has been divided between R1 and R2.

We know that when solving these problems, we look at the data given and thus we can see how we need to manipulate our equations in order to achieve our objective.The following example highlights this point. See to it that you follow the method used and the reasoning behind it.

Part 6: Examples

Figure 4.5: Example 3.1

Figure 4.5 shows a parallel resistive circuit with the following parameters.
${\textstyle I_{s}=5\ {\text{Amps}}}$ ; $R_{1}=2\Omega$ ; $R_{2}=3\Omega$ .

Find: $I_{1},I_{2}$ and $V_{s}$

Solution: from Equation 3.2 we see that.

R_{eq}={\frac {R_{1}R_{2}}{R_{1}+R_{2}}}

Here are the solutions to the above problem:

First Find: $R_{eq}$ : ${\begin{matrix}\ R_{eq}&=&{\frac {(R_{1}\times R_{2})}{(R_{1}+R_{2})}}\\\ \\\ &=&{\frac {(2\times 3)}{(2+3)}}\\\ \\\ R_{eq}&=&1.2\Omega \end{matrix}}$ .

Then;

${\begin{matrix}\ I_{1}&=&I_{s}{\frac {R_{eq}}{R_{1}}}\\\ \\\ &=&(5A){\frac {1.2\Omega }{2\Omega }}\\\ \\\ &=&3A\end{matrix}}$ .

${\begin{matrix}\ I_{2}&=&I_{s}{\frac {R_{eq}}{R_{2}}}\\\ \\\ &=&(5A){\frac {1.2\Omega }{3\Omega }}\\\ \\\ &=&2A\end{matrix}}$ .

${\begin{matrix}\ V_{s}=V_{1}=V_{2}\\\ \\\ V_{2}=I_{2}\times R_{2}\\\ \\\ =2\times 3\\\ \\\ =6Volts\end{matrix}}$ .

Part 7

Do you Remember?

Let's take some time to Reflect on the Material covered thus far. We have learned a great deal about simple resistive circuits and the possible connections they afford us. Here I think you'll want to remember:

Voltage: (V or v - Volts)The electrical potential between two points in a circuit.
Current: (I or i - Amperes)The amount of charge flowing through a part of a circuit.
Power: (W - Watts)Simply P = IV. It is the current times the voltage.
Source: A voltage or current source is the supplier for the circuit.
Resistor: (R measured in Ω - Ohms)A circuit element that "constricts" current flow.

Total Series Resistance: ( $R_{E}=R_{1}+R_{2}\!$ )

Voltage Divider: $V_{1}={\frac {R_{1}\times V_{s}}{R_{1}+R_{2}}}$

Current through resistors connected in series is the same for all resistors.

Two resistors connected in parallel: $R_{eq}={\frac {R_{1}\times R_{2}}{R_{1}+R_{2}}}$

The Current Divider Principle: $I_{1}=I_{s}\times {\frac {R_{eq}}{R1}}$

Do Exercise 4 in Part 8. After being completely satisfied with your work, you can go on to the next page - for the quiz! Good luck :-)

Part 8: Exercise 4

Given 2 resistors $R_{1}=R_{2}=5\Omega$ in parallel, find the $R_{eq}$ .
Given 3 resistors $R_{1}=2\ \Omega$ , $R_{2}=3\ \Omega$ , and $R_{3}=7\ \Omega$ in parallel, and given that the supply current is $15\ A$ , find $R_{eq}$ , $I_{1}$ , $I_{2}$ , $I_{3}$ and the supply voltage $I_{s}$ across these resistors.
Given 4 resistors: $R_{1}=2\Omega$ connected in series to a parallel branch of 3 resistors: $R_{2}=3\Omega$ ; $R_{3}=7\Omega$ and $R_{4}=4\Omega$ Find: total resistance as seen by the voltage source.
Is it possible to effectively connect voltage sources in parallel? If so, what conditions must be met?