Complex Numbers

Complex number representation

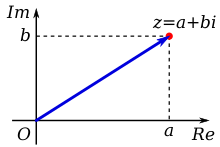
In mathematics, a complex number is an element of a number system that contains the real numbers $\mathbb {R}$ and a specific element denoted $i$, called the imaginary unit, satisfying $i^{2}=-1$. Moreover, every complex number can be expressed in the form $a+bi$, where $a$ and $b$ are real numbers. Because no real number satisfies the above equation, $i$ was called an imaginary number by René Descartes. For the complex number $a+bi$, $a$ is called the real part and $b$ is called the imaginary part. The set of complex numbers is typically denoted by the symbol $\mathbb {C}$. Despite the historical nomenclature "imaginary", complex numbers are regarded in the mathematical sciences as just as "real" as the real numbers and are fundamental in many aspects of the scientific description of the natural world.

Lessons

• Introduction
• From real to complex numbers - (Wiki2Reveal slides)

See Also

• Complex Analysis
• Wikipedia: Complex number
• Wikibooks: Algebra/Complex Numbers
• OpenStax: College Algebra 2e 2.4 - Complex Numbers